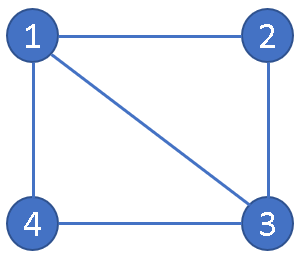
A Graph is a data structure which consists of Vertices and Edges. The Vertices, also referred as Nodes and the edges are the lines that connects any two nodes. For e.g. in the below graph, elements 1, 2, 3, 4 are nodes and lines between 1-2, 2-3, 3-4, 4-1 and 1-3 are edges.
A graph is represented with a notation as G = (V, E) where V is vertices and E are edges.
Contents
Types and properties of graph Applications of graph Graph representation
Below are some important types of graphs.
- Undirected graph:
An undirected graph comprised of set of nodes and edges, and the order of edge that connects two nodes has no direction.
Below is an example of undirected graph.
- Directed graph:
A directed graph comprised of set of nodes and edges, and the order of edge that connects two nodes will represent a direction.
Below is an example of directed graph.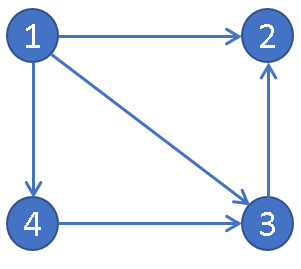
- Weighted graph:
In a weighted graph, an edge that connects nodes will have a value or weight representing the cost of traversing through that path.
Below is an example of directed graph.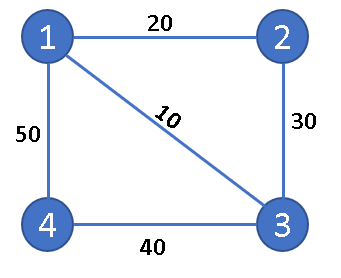
- Finite graph:
A graph will be called as finite graph if the number of vertices and edges in the graph are limited in number.
Below is an example of finite graph.
- Ininite graph:
A graph will be called as infinite graph if the number of vertices and edges in the graph are unknown.
Below is an example of infinite graph.
- Connected graph:
If there is a path between every vertex to all other vertices in the graph is called as connected graph.
Below is an example of connected graph.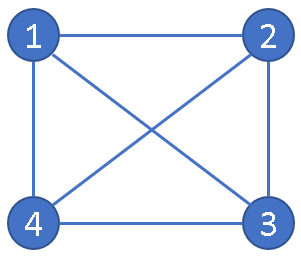
- Disconnected graph:
If there is no path between a vertex and another vertex in the graph that forms disjoint sets in the graph called as disconnected graph.
Below is an example of disconnected graph. As you can see vertex 2 has no path to any other vertices.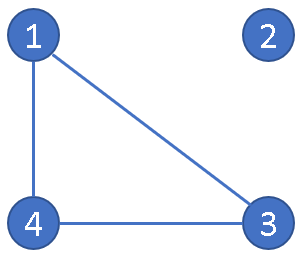
- If a graph contains only one vertex and has no edges is called as Trivial graph.
- If a graph contains multiple vertices, and has no edges is called as Null graph.
- If a graph has relatively few edges than vertices (in general, number of edges are less than |V| log |V|) is called as sparse graph.
- In a graph, if every vertex is connected to other vertex in the graph, is called as Complete graph.
- In a graph, if the number of edges are close the number of edges in a complete graph is called as Dense graph.
-
If a graph contains atleast one graph cycle, is called as Cyclic graph.
In the below example node 1 is connected to 2, 2 is connected to 3, 3 is connected to 4, and 4 is connected back to 1, so it forms a cycle.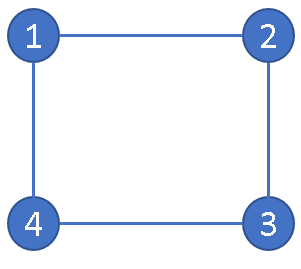
-
If a graph does not contain any cycles, is called as Acyclic graph.
In the below example node 1 is connected to 2, 2 is connected to 3, and 3 is connected to 4.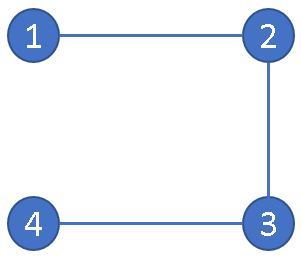
Below are some real world applications of Graphs.
- Geographical map to show how cities connects with roads, flight network and trains network.
- Entitiy relationships in database.
- Social network applications.
- You can model computers network and internet using graphs.
Graph data structure is commonly represented using
- Adjascency matrix.
- Adjascency list.
- Adjascency matrix:
A graph can be represented as adjascency matrix, for example using a 2D array.
For example, consider below undirected graph, as you see nodes that are connected are 1-2 , 2-3, 3-4 and 4-1.
It can be represented as array of arrays, if there is an edge between the nodes, mark that with 1, otherwise 0.
1 2 3 4 1 [0 1 0 1] 2 [1 0 1 0] 3 [0 1 0 1] 4 [1 0 1 0]char[][] graph = { { 0, 1, 0, 1}, { 1, 0, 1, 0}, { 0, 1, 0, 1} { 1, 0, 1, 0} }; - Adjascency list:
A graph can be represented as adjascency list, where each node is represented using a singly linked list, with links to its adjascent nodes if there is an edge.public class Node { private int element; private LinkedList<Node> adjascencyList = new LinkedList<>(); public Node(int element) { this.element = element; } public void addAdjascentNode(Node adj) { adjascencyList.add(adj); } public int getElement() { return element; } public LinkedList getAdjascencyList() { return adjascencyList; } } -
Below code snippet shows how you can create graph nodes and their edges.
Node element_one = new Node(1); Node element_two = new Node(2); Node element_three = new Node(3); Node element_four = new Node(4); // 1-> 2 and 1-> 4 element_one.addAdjascentNode(element_two); element_one.addAdjascentNode(element_four); // 2-> 1 and 2-> 3 element_two.addAdjascentNode(element_one); element_two.addAdjascentNode(element_three); // 3-> 2 and 3-> 4 element_three.addAdjascentNode(element_two); element_three.addAdjascentNode(element_four); // 4-> 3 and 4-> 1 element_four.addAdjascentNode(element_three); element_four.addAdjascentNode(element_one);